sword060's blog

SD Segment Tree Variation, A Shorter And More Efficient Segment Tree
By sword060, history, 17 months ago, In English

Segment Tree is a powerful data structure in programming, that is why it can still be optimized way more. In this blog I will explain one optimization that can make a basic segment tree slightly faster and easier to write. (idea and the code by me)

This does not work on range update range query segment trees.

Introduction:

Let's consider a point update range query segment tree, while querying we visit many of useless Nodes along the way in order to answer the query moving from the root downwards.

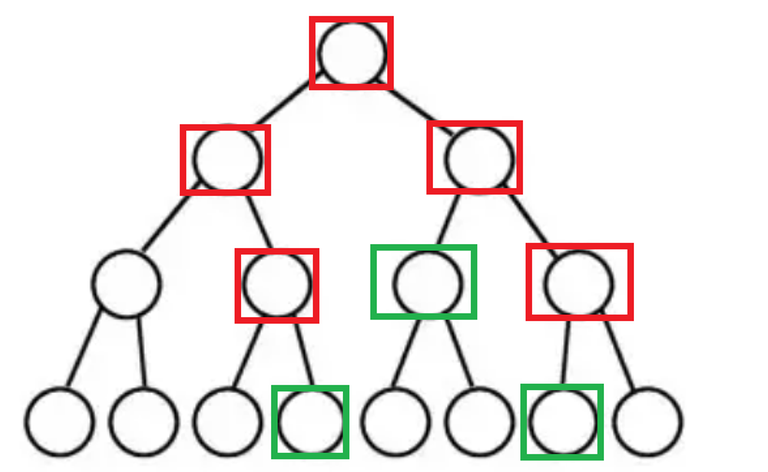
As you can see, there are nodes (marked in red) that are not needed during the recursion, and we only need to visit the important nodes (marked in green).

This is only true when querying in a point update segment tree or updating in a point query segment tree.

Main Idea:

We can solve a query range $$$[l, r]$$$ by noticing we can make it a smaller range $$$[l + X , r]$$$, where $$$X$$$ is any power of two but we need it to be maximum (in order to reduce the time complexity) and these two conditions should be true:

  • $$$l + X - 1 \le r$$$. (We cannot go out of the range)
  • $$$[l, l + X - 1]$$$ is a valid node in the segment tree.
The first condition:
The second condition

At the end, we can solve it now because $$$X$$$ is $$$2$$$ power the minimum between $$$log_2(r-l+1)$$$ and $$$log_2( M \& -M )$$$ because it satisfies the first and second conditions and is the maximum value possible.

C++ Code:

We can preprocess $$$log_2(K)$$$ for each $$$1 \le K \le N$$$ in an array.

Note that this only works when $$$N$$$ (the number of leaves) is a power of 2.

At each step we calculate the size of the movement $$$X$$$ which is equal to $$$2^K$$$

The following codes calculate sum in the range $$$L$$$ to $$$R$$$, assuming the segment tree is built after possibly several update queries.

Recursive:

long long query(int l, int r){
	if(l > r)return 0;
	int node = N + l - 1;
	int K = min(logs[node & -node], logs[r - l + 1]);
	return (query(l + (1 << K), r) + seg[node >> K]);
}

Iterative:

long long query(int l, int r){
	long long ret = 0;
	while(l<=r){
		int node = N + l - 1;
		int K = min(logs[node & -node], logs[r - l + 1]);
		ret = (ret + seg[node >> K]);
		l += (1 << K);
	}
	return ret;
}

This can also be applied to range update point query segment trees:

void update(int l, int r, int val){
	while(l<=r){
		int node = N + l - 1;
                int K = min(logs[node & -node], logs[r - l + 1]);
		seg[node >> K] += val;
		lazy[node >> K] += val;
		l += (1 << K);
	}
}

Benchmark:

Test-Cases Generator
SD-Segment-Tree Code
Iterative-Segment-Tree Code
Recursive-Segment-Tree Code
Size of the array and the number of queries Time of SD-Segment-Tree /S Time of Recursive-Segment-Tree /S Time of Iterative-Segment-Tree /S
$$$N,Q = 2^{16}$$$ 00.2847 00.3163 00.2292
$$$N,Q = 2^{17}$$$ 00.4311 00.5335 00.4414
$$$N,Q = 2^{18}$$$ 00.8322 00.9534 00.9729
$$$N,Q = 2^{19}$$$ 01.9915 02.1086 01.6837
$$$N,Q = 2^{20}$$$ 03.6747 04.4253 03.7347
$$$N,Q = 2^{21}$$$ 08.0204 08.6896 07.7844
$$$N,Q = 2^{22}$$$ 20.9266 27.0589 24.3542
$$$N,Q = 2^{23}$$$ 50.0656 61.9385 49.8065

Conclusion:

This variation has the same time complexity as the normal segment tree $$$O(log(N))$$$ per query, but might need more memory if you preprocess Logs array.

The constant factor is smaller because of the unnecessary nodes we don't visit but in practice the time it takes is not significant than the normal segment tree for smaller array sizes.

This can only be useful for squeezing in time limits or for becoming an easier way to implement segment trees because it is shorter.

UPD: Added Benchmark

  • Vote: I like it
  • +123
  • Vote: I do not like it

Comments Comments (11)
| Write comment?
»
17 months ago, # |
  Vote: I like it +58 Vote: I do not like it

Just don't preprocess the logs.

In c++ you can do __lg(x&-x) or use __builtin_ctz(x). I compared them with preprocessing logs many times in alot of problems (most of cases is implementing sparse table) but preprocessing was never faster.

→ Reply
»
17 months ago, # |
  Vote: I like it -13 Vote: I do not like it

Isn't this idea very similar to BIT? What are the advantages of this segment tree compared to BIT?

→ Reply
  • »
    »
    17 months ago, # ^ |
      Vote: I like it +55 Vote: I do not like it

    It's hard to do the range min query with BIT. BIT is usefull in sum/xor only.

    I will understand if you asked what is the diffrence between this and the known bottom up segtree, but BIT isn't compared to segtree.

    → Reply
    • »
      »
      »
      17 months ago, # ^ |
        Vote: I like it +13 Vote: I do not like it

      Recursive is often used instead of iterative segment trees because it is easier (although it is slower), this variation might also not be faster than iterative but it is much easier to implement and seems more intuitive. Most of the times just any segment tree you use works so its a matter of what you like more.

      → Reply
  • »
    »
    17 months ago, # ^ |
      Vote: I like it +36 Vote: I do not like it

    BIT queries only on a prefix of the array, Segment trees query on subarrays
    You can't solve subarray queries using BIT if the merging operation doesn't have an inverse (like Max/Min)

    Not to mention also that lazy propagation is exclusive to segment trees

    → Reply
    • »
      »
      »
      17 months ago, # ^ |
        Vote: I like it +23 Vote: I do not like it

      This is an RMQ BIT, but it's an overkill anyway Haven't seen anyone using it

      → Reply
»
17 months ago, # |
  Vote: I like it +9 Vote: I do not like it

Brilliant, thx for sharing :)

→ Reply
»
17 months ago, # |
  Vote: I like it +58 Vote: I do not like it

HD Segment Tree When

→ Reply
»
17 months ago, # |
← Rev. 2   Vote: I like it +19 Vote: I do not like it

Deleted, because i was wrong.

→ Reply
»
2 weeks ago, # |
← Rev. 4   Vote: I like it +5 Vote: I do not like it

here's maybe some subtle benefit of this style of segment tree:

Let's say you want to templatize a point-update segment tree. You can use KACTL https://github.com/kth-competitive-programming/kactl/blob/main/content/data-structures/SegmentTree.h . But then I came across this problem https://mirror.codeforces.com/contest/1814/problem/E where I couldn't figure out what unit should be.

So I thought for a while about how to code a templatized point-update segment tree where you don't need to pass in a unit. And this blog is a way to do it: as it describes how to repeatedly get the first node in range, so you can initialize the query result to the first node instead of to unit.

295168090

→ Reply
  • »
    »
    2 weeks ago, # ^ |
      Vote: I like it +10 Vote: I do not like it

    You can always define the unit as some sentinel value, and adapt the merge function to handle it accordingly, in these (rare) cases where you don't know what the unit might be.

    → Reply